On the edge of a horizontal platform stands a man of mass 80 kg. The platform is a round homogeneous disk with mass 160 kg, rotating around a vertical axis, passing through its center, with a frequency 6 rpm. How many revolutions per minute will the platform make if the person moves from the edge of the platform to its center? Calculate the moment of inertia as for a material point.

This task was posted by visitors in the section We decide together September 19, 2007.

Solution:

The "man-platform" system is closed in the projection onto the axis Y, because moments of forces M m 1 g = 0 and M m 2 g = 0 to this axis. Therefore, you can use the law of conservation of angular momentum. In the projection on the axis Y:

We solve the last equation for the unknown frequency of rotation of the "platform-man" n 2:

After calculations: n 2 \u003d 0.2 (r / s) \u003d 12 rpm. The task is a university one and is solved here at the request of visitors as an exception.

3.41. What work A does a person do when moving from the edge of the platform to its center under the conditions of the previous task? Platform radius R = 1.5 m.

3.42. A horizontal platform with a mass m = 80 kg and a radius R = 1 m rotates with a frequency n, = 20 rpm. A man stands in the center of the platform and holds weights in his outstretched hands. With what frequency n2 will the platform rotate if a person, lowering his hands, reduces his moment of inertia from J1 = 2.94 to J2 = 0.98 kg m2? Treat the platform as a homogeneous disk.

3.43. How many times did it increase kinetic energy platforms with a person in the conditions of the previous task?

3.44. A person with mass m0 = 60 kg is on a fixed platform with mass m = 100 kg. With what frequency n will the platform rotate if a person moves in a circle with a radius r = 5 m around the axis of rotation? Human movement speed relative to the platform v0 = 4 km/h. Platform radius R = 10m. Consider the platform as a homogeneous disk, and the person as a point mass.

3.45. A homogeneous rod with a length l = 0.5 m makes small oscillations in the vertical plane about the horizontal axis passing through its upper end. Find the oscillation period T of the rod.

A task: A horizontal platform rotates uniformly about a vertical axis passing through its center. At a distance equal to a third of the radius of the platform, a small body breaks away from its surface and slides along it without friction. How long will it take for the body to fly off the platform if it was moving with an acceleration of 0.1 m/s^2 before it took off? Platform radius 60 cm.

Solution:

Let's denote a - the acceleration of the body, R - the radius of the platform, t - the time after which the body will fly off the platform, v - the linear velocity of the body on the platform, S - the path that the body will pass.

To make it easier to imagine the movement of the body on the platform, let's make a drawing (Fig. 15). Let's look at the platform from above and draw a circle, show its center O and draw a horizontal radius R. Then, at a distance equal to a third of the radius from the edge of the platform, draw the body at point M at the moment of separation. This means that at this moment the distance from the body to the center of the platform was two-thirds of the radius.

Now let's think. We know the acceleration of the body a before taking off from the surface of the platform. But the platform rotates uniformly, which means that this is its centripetal acceleration. At the moment of separation, the linear velocity of the body v is directed tangentially to the circle along which it moved before separation. The radius of this circle was
(2/3)R . And we know the formula that relates linear velocity to centripetal acceleration. Applied
for our task, it will look like this:

After separation, the body will move to the edge of the platform without friction. This means that this movement will be uniform and rectilinear with a speed v. Then the body will fly off the platform at point C, having traveled the path S. If this path is divided by the linear velocity of the body, we will find the desired time t, after which the body will fly off the platform:

The further course of the decision is clear. We find the path S from the right triangle MCO using the Pythagorean theorem, and the linear velocity v from expression (1), and we substitute all this into equality (2). Let's get started. According to the Pythagorean theorem

Now from (1) we find the linear velocity v:

It remains for us to substitute the right-hand sides of equalities (3) and (4) into formula (2), and the problem in general view will be resolved. We substitute:

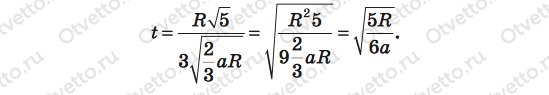
The problem is generally solved. Plug in the numbers and calculate. 60 cm = 0.6 m.

Answer: 2.2 c.